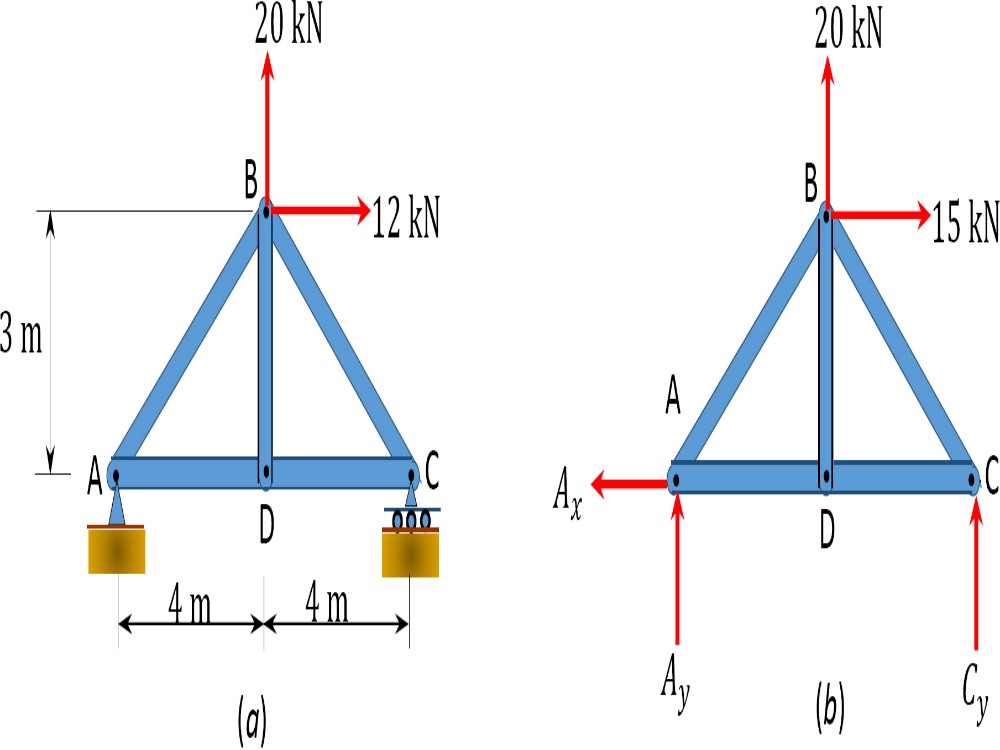
We are ready to start talking business, to buy a loaf of bread. Up until now we have focused on the rudimentary basics of the language; the vocabulary of force, moment, couple and the syntax of static equilibrium of an isolated particle or extended body. This has been an abstract discourse for the most part. We want now to start speaking about “extended bodies”as structural members, as the building blocks of truss structures, frame structures, shafts and columns and the like. We want to be go beyond questions about forces and moments required to satisfy equilibrium and ask “…When will this structure break? Will it carry the prescribed loading?” We will discover that, with our current language skills, we can only answer questions of this sort for one type of structure, the truss structure, and then only for a subset of all possible truss structures.

To go further we will need to broaden our scope, beyond the requirements of force and moment equilibrium, and analyze the deformations and displacements of extended bodies in order to respond to questions about load carrying ability regardless of the type and complexity of the structure at hand – the subject of a subsequent chapter. Here we will go as far as we can go with the vocabulary and rules of syntax at our disposal. After all, the requirements of force and moment equilibrium still must be satisfied whatever structure we confront. A truss structure is designed, fabricated, and assembled such that its members carry the loads in tension or compression. More abstractly, a truss structure is made up of straight, two-force members, fastened together by frictionless pins; all loads are applied at the joints. Now, we all know that there is no such thing as a truly frictionless pin; you will not find them in a suppliers catalogue. And to require that the loads be applied at the joints alone seems a severe restriction. How can we ensure that this constraint is abided by in use? We can’t and, indeed, frictionless pins do not exist.

This is not to say that there are not some ways of fastening members together that act more like frictionless pins than other ways. What does exist inside a truss structure are forces and moments of a quite general nature but the forces of tension and compression within the straight members are the most important of all if the structure is designed, fabricated, and assembled according to accepted practice.

Any member of the structure shown above will, in our abstract mode of imagining, be in either tension or compression – a state of uniaxial loading. Think of having a pair of special eyeglasses – truss seeing glasses – that, when worn, enable you to see all members as straight lines joined by frictionless pins and external forces applied at the joints as vectors. This is how we will usually sketch the truss structure, as you would see it through such magical glasses. Now if you look closer and imagine cutting away one of the members, say with circular cross-section, you would see something like what’s shown: This particular member carries its load, F, in tension; The member is being stretched.