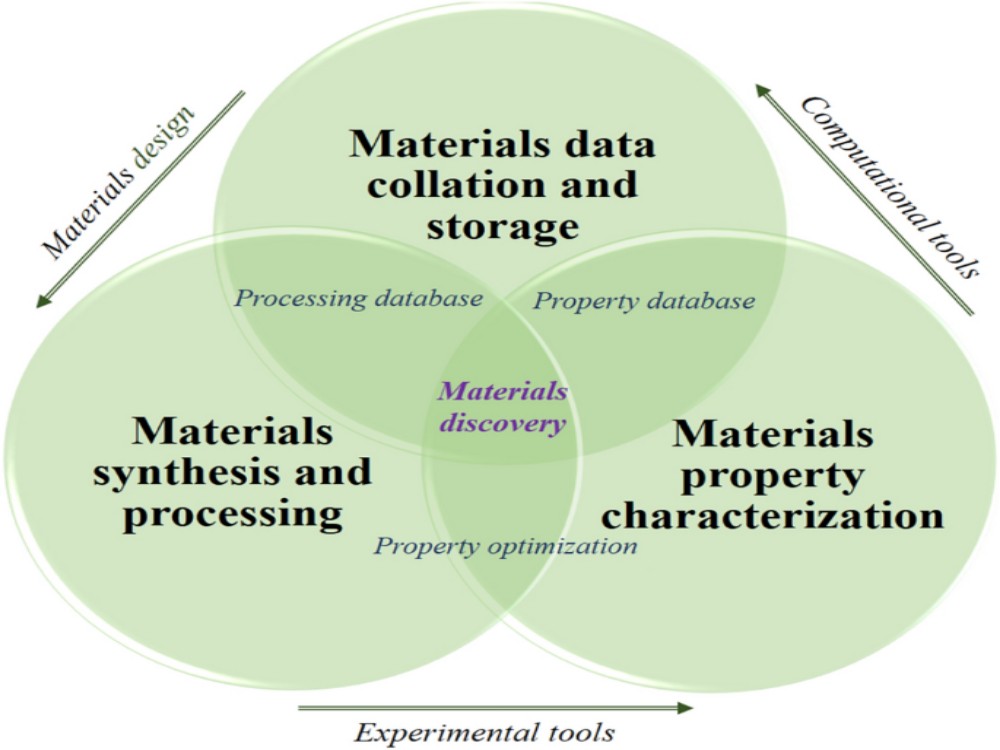
In thermodynamic terminology, a state of purely elastic material response corresponds to an equilibrium state, and a process during which there is purely elastic response corresponds to a sequence of equilibrium states and hence to a reversible process. The second law of thermodynamics assures that the heat absorbed per unit mass can be written θds, where θ is the thermodynamic (absolute) temperature and s is the entropy per unit mass. Hence, writing the work per unit volume of reference configuration in a manner appropriate to cases when infinitesimal strain can be used, and letting ρ0 be the density in that configuration, from the first law of thermodynamics it can be stated that ρ0θds + tr([σ][dε]) = ρ0de, where e is the internal energy per unit mass. This relation shows that if e is expressed as a function of entropy s and strains [ε], and if e is written so as to depend identically on εij and εji, then σij = ρ0∂e([ε], s)/∂εij.

Alternatively, one may introduce the Helmholtz free energy f per unit mass, where f = e − θs = f([ε], θ), and show that σij = ρ0∂f([ε], θ)/∂εij. The latter form corresponds to the variables with which the stress-strain relations were written above. Sometimes ρ0f is called the strain energy for states of isothermal (constant θ) elastic deformation; ρ0e has the same interpretation for adiabatic (s = constant) elastic deformation, achieved when the time scale is too short to allow heat transfer to or from a deforming element. Since the mixed partial derivatives must be independent of order, a consequence of the last equation is that ∂σij([ε], θ)/∂εkl = ∂σkl([ε], θ)/∂εij, which requires that Cijkl = Cklij, or equivalently that the matrix [c] be symmetric, [c] = [c]T, reducing the maximum possible number of independent elastic constraints from 36 to 21. The strain energy W([ε]) at constant temperature θ0 is W([ε]) ≡ ρ0f([ε], θ0) = (1/2){ε}T[c]{ε}.

The elastic moduli for adiabatic response are slightly different from those for isothermal response. In the case of the isotropic material, it is convenient to give results in terms of G and K, the isothermal shear and bulk moduli. The adiabatic moduli G and K̄ are then G = G and K̄ = K(1 + 9θ0K α2/ρ0cε), where cε = θ0∂s([ε],θ)/∂θ, evaluated at θ = θ0 and [ε] = [0], is the specific heat at constant strain. The fractional change in the bulk modulus, given by the second term in the parentheses, is very small, typically on the order of 1 percent or less, even for metals and ceramics of relatively high α, on the order of 10−5/kelvin.

The fractional change in absolute temperature during an adiabatic deformation is found to involve the same small parameter: [(θ − θ0)/θ0]s = const = −(9θ0Kα2/ρ0cε) [(ε11 + ε22 + ε33)/3αθ0]. Values of α for most solid elements and inorganic compounds are in the range of 10−6 to 4 × 10−5/kelvin; room temperature is about 300 kelvins, so 3αθ0 is typically in the range 10−3 to 4 × 10−2. Thus, if the fractional change in volume is on the order of 1 percent, which is quite large for a metal or ceramic deforming in its elastic range, the fractional change in absolute temperature is also on the order of 1 percent. For those reasons, it is usually appropriate to neglect the alteration of the temperature field due to elastic deformation and hence to use purely mechanical formulations of elasticity in which distinctions between adiabatic and isothermal response are neglected.