In Florentine Italy in the seventeenth century, well diggers observed that when they used suction pumps, water would not rise more than about 10 m. In 1642 they came to the famous Galileo for help, but he did not want to be bothered. As an alternative, they sought the help of Torricelli. He learned from experiments that water was not being pulled up by the vacuum but rather was being pushed up by the local air pressure.
Therefore, the maximum height that water could be raised from a well depended on the atmospheric pressure. Pressure is defined as “the normal (perpendicular) force per unit area.” In the SI system the force is expressed in newtons and the area in square meters; then the pressure is N/m2 or pascal (Pa). (The value of a pascal is so small that the kilopascal (kPa) is a more convenient unit of pressure.) In the AE system the force is the pounds force and the area used is square inches.
What are other units for pressure?
Look at Table 2.6 for some of the most common ones. Note that each pressure unit is expressed as the equivalent of 1 standard atmosphere (atm).

Keep in mind that the pounds referred to in psi are pounds force, not pounds mass, so the cartoon caption “It says inflate to 12 pounds. How can I throw a 12-pound football?” causes a chuckle. Examine Figure 2.3. Pressure is exerted on the top of the mercury in the cylinder by the atmosphere. The pressure at the bottom of the column of mercury is equal to the pressure exerted by the mercury plus that of the atmosphere on the mercury.
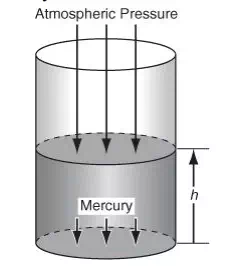
The pressure at the bottom of the static (nonmoving) column of mercury (also known as the hydrostatic pressure) exerted on the sealing plate is
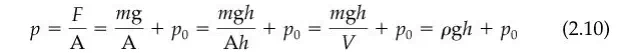
where the first term after the pressure p is the definition of pressure, the second term is the combination of atmospheric pressure and the pressure change due to the column of liquid, the third shows how h can be added to the numerator and denominator to get the volume in the denominator, in the fourth term volume is substituted for area times height, and in the fifth term the density is substituted for mass divided by volume. The notation used is as follows:

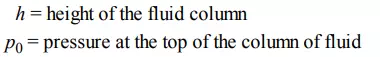
You can calculate the force exerted at the bottom of a static fluid by applying Equation (2.10). For example, suppose that the cylinder of fluid in Figure 2.3 is a column of mercury with a crosssectional area of 1 cm2 and is 50 cm high. From Table D.1 in Appendix D you can find that the specific gravity of mercury at 20°C, and hence the density of the Hg, is 13.55 g/cm3 . Thus, the force exerted by the mercury alone on the 1 cm2 section of the bottom plate by the column of mercury is
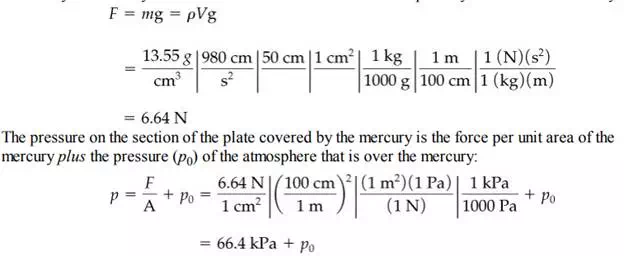
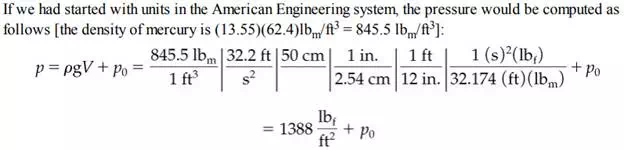
Sometimes in engineering practice, a liquid column is referred to as head of liquid, the head being the height of the column of liquid. Thus, the pressure of the column of mercury could be expressed simply as 50 cm Hg, and the pressure on the sealing plate at the bottom of the column would be 50 cm Hg + p0