The Schrödinger equation, sometimes called the Schrödinger wave equation, is a partial differential equation. It uses the concept of energy conservation (Kinetic Energy + Potential Energy = Total Energy) to obtain information about the behavior of an electron bound to a nucleus. It does this by allowing an electron’s wave function, Ψ, to be calculated.
Solving the Schrödinger equation gives us Ψ and Ψ2. With these we get the quantum numbers and the shapes and orientations of orbitals that characterize electrons in an atom or molecule.
The Schrödinger equation gives exact solutions only for nuclei with one electron: H, He+, Li2+, Be3+, B4+, C5+, etc. In mathematical language, we say that analytic solutions for Ψ are possible only for one-electron systems. One-electron systems are often described as hydrogenic – meaning “like hydrogen.”
For all other atoms, ions, and molecules, no analytic solutions for Ψ are possible; approximation methods of calculation, such as the orbital approximation and variation theorem, are then utilized.
There is a time-dependent Schrödinger equation and a time-independent Schrödinger equation.
The time-independent equation considers the electron’s quantum state to be unchanging, hence it considers the electron as a standing wave
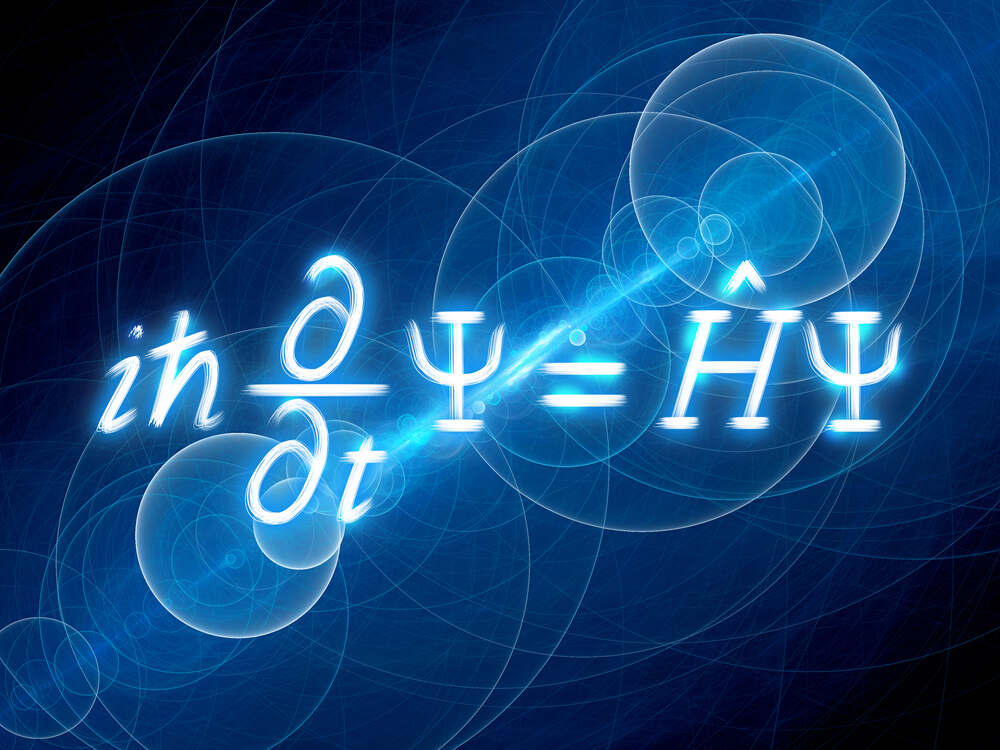
Comments are closed