You can hardly go through a single day without noticing or hearing what the temperature is. Believe it or not, considerable controversy exists among some scientists as to what the correct definition of temperature is (consult some of the references at the end of this chapter for further information). Some scientists prefer to say that temperature is a measure of the energy (mostly kinetic) of the molecules in a system.

Other scientists prefer to say that temperature is a property of the state of thermal equilibrium of the system with respect to other systems because temperature tells us about the capability of a system to transfer energy (as heat). In this book we use four classes of temperature measures, two based on a relative scale, degrees Fahrenheit (°F) and Celsius (°C), and two based on an absolute scale, degrees Rankine (°R) and kelvin (K).

Relative scales are the ones you hear the TV or radio announcer give and are based on a specified reference temperature (32°F or 0°C) that occurs in an ice-water mixture (the freezing point of water). Absolute temperature scales have their zero point at the lowest possible temperature that we believe can exist. As you may know, this lowest temperature is related both to the ideal gas laws and to the laws of thermodynamics.

The absolute scale that is based on degree units the size of those in the Celsius scale is called the kelvin scale (in honor of Lord Kelvin, 1824–1907); the absolute scale that corresponds to the Fahrenheit degree units is called the Rankine scale (in honor of W. J. M. Rankine, 1820–1872, a Scottish engineer). Figure 2.2 illustrates the relationships between relative temperature and absolute temperature. We shall usually round off absolute zero on the Rankine scale of –459.67°F to –460°F; similarly, –273.15°C frequently will be rounded off to –273°C. Remember that 0°C and its equivalents are known as standard conditions of temperature.

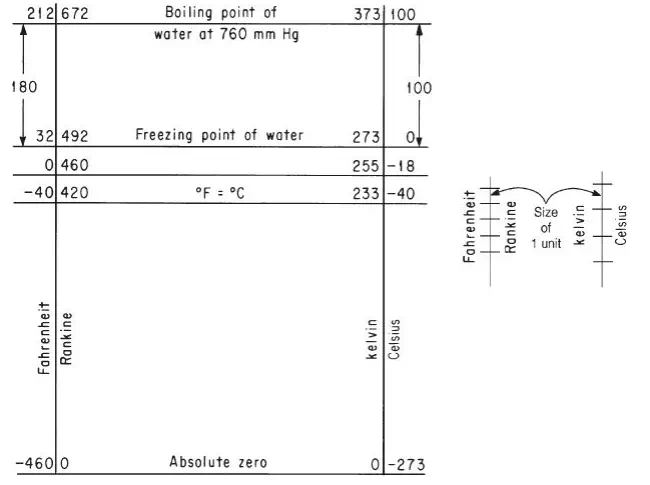
Now we turn to a topic that causes endless difficulty in temperature conversion because of confusing semantics and notation. To start, you should recognize that the unit degree (i.e., the unit temperature difference or division) on the kelvin-Celsius scale is not the same size as that on the RankineFahrenheit scale. If we let Δ°F represent the unit temperature difference on the Fahrenheit scale and Δ°R be the unit temperature difference on the Rankine scale, and Δ°C and Δ K be the analogous units in the other two scales, you probably are aware that