The confines in which chemical reactions occur are called reactors. A reactor can be a chemical reactor in the traditional sense or other entities, for example, a chemical vapor deposition apparatus for making computer chips, an organ of the human body, and the atmosphere of a large city. In this chapter, the discussion of reactors is limited to topics germane to the determination of reaction rates. Later in this text, strategies for attacking the problems of mathematically describing and predicting behavior of reactors in general are presented. In practice, conditions in a reactor are usually quite different than the ideal requirements used in the definition of reaction rates.
Normally, a reactor is not a closed system with uniform temperature, pressure, and composition. These ideal conditions can rarely if ever be met even in experimental reactors designed for the measurement of reaction rates. In fact, reaction rates cannot be measured directly in a closed system. In a closed system, the composition of the system varies with time and the rate is then inferred or calculated from these measurements. There are several questions that can be put forth about the operation of reactors and they can be used to form the basis of classifying and defining ideal conditions that are desirable for the proper measurements of reaction rates.
The first question is whether the system exchanges mass with its surroundings. If it does not, then the system is called a batch reactor. If it does, then the system is classified as a flow reactor. The second question involves the exchange of heat between the reactor and its surroundings. If there is no heat exchange, the reactor is then adiabatic. At the other extreme, if the reactor makes very good thermal contact with the surroundings it can be held at a constant temperature (in both time and position within the reactor) and is thus isothermal.
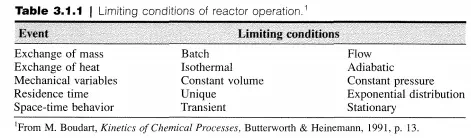
The third question concerns the mechanical variables: pressure and volume. Is the reactor at constant pressure or constant volume? The fourth question is whether the time spent in the reactor by each volume element of fluid is the same. If it is not the same, there may exist a distribution ofresidence times and the opposite extreme of a unique residence time is an exponential distribution.
The fifth question focuses on a particular fixed volume element in the reactor and whether it changes as a function of time. If it does not, then the reactor is said to operate at a stationary state. If there are time variations, then the reactor is operating under transient conditions. A nontrivial example of the transient situation is designed on purpose to observe how a chemically reactive system at equilibrium relaxes back to the equilibrium state after a small perturbation.
This type of relaxation experiment can often yield informative kinetic behavior. The ten possibilities outlined above are collected in Table 3.1.1. Next, ideal reactors will be illustrated in the contexts of the limiting conditions of their operation.