The equipartition theorem, also known as the law of equipartition, equipartition of energy or simply equipartition, states that every degree of freedom that appears only quadratically in the total energy has an average energy of ½kBT½kBT in thermal equilibrium and contributes ½kB½kB to the system’s heat capacity. Here, kBkB is the Boltzmann constant, and T is the temperature in Kelvin. The law of equipartition of energy states that each quadratic term in the classical expression for the energy contributes ½kBT to the average energy. For instance, the motion of an atom has three degrees of freedom (number of ways of absorbing energy), corresponding to the x, y and z components of its momentum. Since these momenta appear quadratically in the kinetic energy, every atom has an average kinetic energy of 3/2kBT3/2kBT in thermal equilibrium. The number of degrees of freedom of a polyatomic gas molecule is 3N3N where NN is the number of atoms in the molecule. This is equal to number of coordinates for the system; e.g. for two atoms you would have x, y, z for each atom.
Translations
The translational contribution to the average energy is derived in terms of the derivative of the translational partition:

Introducing the translational partition function derived earlier, Equation 11 becomes

Thus, the three translational degrees of freedom in three dimensions satisfy the equipartition theorem with each translational degree providing ½kBT½kBT of energy.
Rotations
Consider the molecular partition functions. The average rotational energy to the average energy is derived in terms of the derivative of the translational partition:
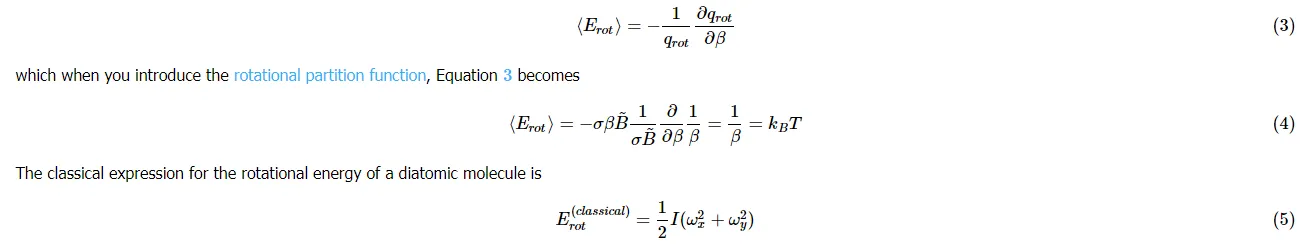
where II is the moment of inertia and ωxωx and ωyωy are the angular velocities in the xx and yy directions. The rotation along the molecular axis (the zz axis here) has no meaning in quantum mechanics because the rotations along the molecular axis lead to configurations which are indistinguishable from the original configuration. The two rotational degrees of freedom have thus given a value of kTkT with with each rotational degree providing ½kBT½kBT of energy.
Vibrations
Consider vibrational motions. The average vibrational energy to the average energy is derived in terms of the derivative of the translational partition:
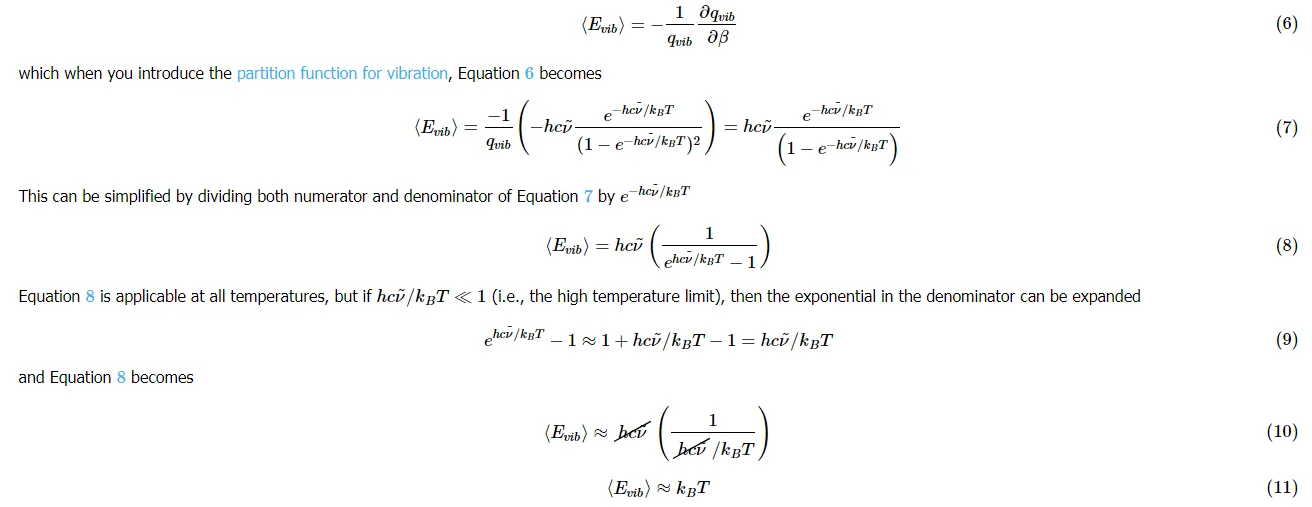
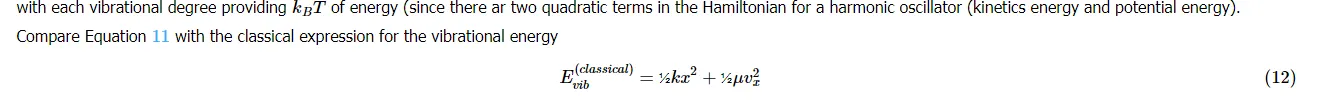
At high temperature the equipartition theorem is valid, but at low temperature, the expansion in Equation 99 fails (or more terms are required). In this case, only a few vibrational states are occupied and the equipartition principle is not typically applicable.
Heat Capacity
Heat capacity at constant volume CvCv, is defined as

The equipartition theorem requires that each degree of freedom that appears only quadratically in the total energy has an average energy of ½kBT in thermal equilibrium and, thus, contributes ½kB to the system’s heat capacity. Thus the three translational degrees of freedom each contribute ½R to (3/2 R). The contribution of rotational kinetic energy will be R for the linear, and 3/2R for the nonlinear molecules. For the vibration, an oscillator has quadratic kinetic and potential terms, making the contribution of each vibrational mode R. However, kBT has to be much greater than the spacing between the quantum energy levels. If this is not satisfied, the heat capacity will be reduced and which drop to zero at low temperatures. The corresponding degree of freedom is said to be frozen out; this is the situation for the vibrational degrees of freedom at room temperature and that is why the usual assumption is that they will not contribute.
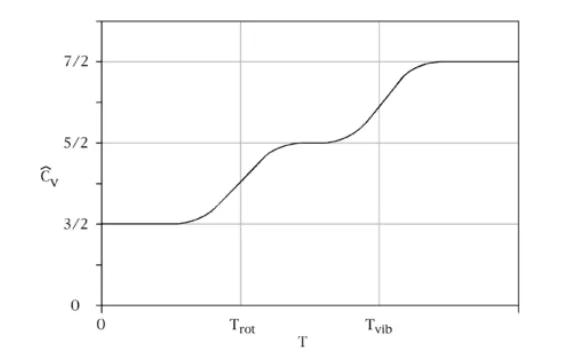
Figure 11: Idealized plot of the molar specific heat of a diatomic gas against temperature. It agrees with the value (7/2)R predicted by equipartition at high temperatures (where R is the gas constant), but decreases to (5/2)R and then (3/2)R at lower temperatures, as the vibrational and rotational modes of motion are “frozen out”. The failure of the equipartition theorem led to a paradox that was only resolved by quantum mechanics. For most molecules, the transitional temperature Trot is much less than room temperature, whereas Tvib can be ten times larger or more. A typical example is carbon monoxide, CO, for which Trot ≈ 2.8 K and Tvib ≈ 3103 K. For molecules with very large or weakly bound atoms, Tvib can be close to room temperature (about 300 K); for example, Tvib ≈ 308 K for iodine gas, I2. Image used with permission (Public domain; PD-Self)